BMPT "Terminator"

Very simple reason for my suggestion. The turret with 2A72 guns will have much lower recoil so far less vibrations than the turret with 2A42 guns. This then leads to more firing accuracy finally. Additionally, the 2A72 gun is cheaper to aquire and maintain.

When the feeding limitations to be solved,  the total rof of 2 2A72 guns is 1,100 rpm that is far enough to deal with its assigned targets.

PS: The problem in your comments is you just "hear" some where without facts and figures. That is the reason why you still keep thinking that the 6KN recoil 2A72 gun is not suitable than the 40KN recoil 2A42 gun for ligh vehicles.

Fact of the matter is that the "Epoch" turret with a 2A42 is considered the standard unmanned turret for the Kurganets, Bumerang and T-15. The 2A72 is only being used on some specific vehicles. Replacing the 2A42s on a BMPT with 2A72s won't improve its accuracy by any amount due to "less vibrations", not only because it's a heavy vehicle with a large unmanned turret, but because the mechanical accuracy of long recoil-operated cannon is inherently less than a gas operated cannon, even if you brace the barrel with a rigid support.

Less recoil force is obviously desirable, but it doesn't mean that the cannon will be more accurate if the barrel wobbles like a plastic ruler every time you fire it. This is much more nuanced than you think, so please try to be polite and stop pretending to know everything.

You need to respect people first before you ask back for their polite.

Your comments still lack facts and figures and most of things are your owned assumptiond. Those vehicles are strong enough, remotely controlled and have only one gun so the problems of strong recoil and leaking smock of 2A42 are not big issues compare with what they gain from its high rate of fire.

I know the 2A42 gun is dual-feed, can be feeded 2 type of rounds parallel. However as mentioned in previous posts, the guns on BMPT-72 can be feeded only 1 type of rounds instead of 2. This means either:

- 1 gun is feeded HEI and the other is feeded API.
- Or both of guns are feeded HEI.
- Or both of guns are feeded API.

Which begs the obvious questions of why... why mount a dual feed gun in a turret with single feed only... the external gun mount means fumes from firing are irrelevant so there is no reason not to use the 2A42... it is a good solid reliable gun which is resistant to dust and dirt...

The HEI and APT rounds the BMPT fires both have projectiles in the 0.4kg weight range (.390 and .4 respectively) and fire them at similar speeds (960m/s and 970m/s) so the ballistics of each round would be very similar so it would not be impossible to have both guns firing a mixed belt of both types of rounds... making dual feed redundant...

Is it possible that the recoil operated firing mechanism of the 2A72's becomes less suitable when firing both cannons simultaneously?

kopyo-21 wrote:You need to respect people first before you ask back for their polite.

Point to where I insulted you.

kopyo-21 wrote:
Your comments still lack facts and figures and most of things are your owned assumptiond.

Your "facts and figures" are from the Weaponsystems.net website:

"Recoil force" for 2A42: 30-40 kN

"Recoil force" for 2A72: 6 kN


The Tulamash website says:

Recoil force for 2A42: 40-50 kN

Recoil force for 2A72: 60 kN

Somebody made a mistake here. Do you wanna make a bet?  

BTW the same information is repeated in the technical manual and user's manual for the 2A42 and 2A72. I have both, and it says the same thing. Recoil force of 2A42 is 40-50 kN (4000-5000 kgf), and recoil force of 2A72 is 60 kN (6000 kgf).

kopyo-21 wrote:Those vehicles are strong enough, remotely controlled and have only one gun so the problems of strong recoil and leaking smock of 2A42 are not big issues compare with what they gain from its high rate of fire.

That doesn't explain why the Vikhr UGV exists, or why the BMD-2 uses a 2A42, or why the new low silhouette turrets designed for the BTR-80 have a 2A42.

No one know it all so don't judge "meaningless" carelessly about other comments, especially when you have not really understood all what they say.

If you know about the differences beetwen the gas-operated guns (like 2A42) and recoil-operated guns (like 2A72), you will know what does the recoil 60KN of 2A72 mean.

The guns use long-recoil to operate so it neede enough the recoil force to operate the gun's mechanism instead of eliminating it. That is the reason why we have never ever seen it with muzzle brake like 2A42 and other guns for reducing the recoil force. When firing, most of 60KN recoil force will be used to power the gun system so the recoil left 6KN on the turret finally (as my source mentioned)

In the opposite way, the 2A42 guns mainly use the gas from the cartridge power burning to operate the gun system. When firing Its recoil according to Tula's is 50-60KN. The 30-40KN as mt source mentioned should be the recoil left on the turret finally.

Besides bigger and much stronger recoil, one more thing important about 2A42. Its design make it be installed a little bit off right of turret axis. When firing at fast mode and long busts, it makes the turret spin and of cource, firing inaccuracy.

"The autocannon is mounted slightly off to the right, which causes an unfortunate tendency for the turret to be pushed slightly to the right when the autocannon fires bursts in the high rate of fire mode. The effect is totally negligible if the gunner uses short bursts, so this idiosyncrasy shouldn't pose any harmful effects to accuracy when accuracy matters most. However, the huge recoil forces generated even when firing in the low rate of fire is a tremendous burden on the horizontal stabilizer if the turret were spinning while firing. "

and

"This problem possibly led to the use of the  low-recoil 2A72 cannon in the BPPU-1 turret for the BTR-80A and the BTR-82A instead of the 2A42."

https://thesovietarmourblog.blogspot.com/2016/05/bmp-2.html?m=1
https://thesovietarmourblog.blogspot.com/2015/10/?m=1

GarryB wrote:Which begs the obvious questions of why... why mount a dual feed gun in a turret with single feed only... the external gun mount means fumes from firing are irrelevant so there is no reason not to use the 2A42... it is a good solid reliable gun which is resistant to dust and dirt...

The HEI and APT rounds the BMPT fires both have projectiles in the 0.4kg weight range (.390 and .4 respectively) and fire them at similar speeds (960m/s and 970m/s) so the ballistics of each round would be very similar so it would not be impossible to have both guns firing a mixed belt of both types of rounds... making dual feed redundant...

It is not about the FCS as both rounds have the same ballistic.

My point is the HEI rounds content ~ 33 grams of HE while the API's is 0. Therefore if you use the API to attake the troops or other soft targets, its effective is not more than a 7.62mm round or just make the hole bigger.

The same if you attake the heavily amored targets by HEI round, they are harmless and wasteful.

It is not about the FCS as both rounds have the same ballistic.

If they have the same ballistics then they can be fired in mixed round belts so dual feed becomes redundant.

My point is the HEI rounds content ~ 33 grams of HE while the API's is 0. Therefore if you use the API to attake the troops or other soft targets, its effective is not more than a 7.62mm round or just make the hole bigger.

Actually if you are paying attention the I in HEI and API mean the same thing... incendiary.... material that starts fires.

If the target is a building the HEI rounds will just splatter the outer walls, while the API will punch holes through the walls and start fires inside and kill those inside.

Both would compliment each other.

Of if what you are saying is true and these dual fed weapons are firing different rounds with different trajectories then one would expect the gun elevation to be different, or both guns would never fire at one time.... because as you say you would never need both API and HEI rounds for the same target...

Against armoured targets HEI rounds can still damage optics and external equipment and also injure people nearby.

Both the 2A42 and 2A72 use the fired shot to reload... the 2A72 uses a long recoil stroke with the barrel and bolt locked together so by the time they recoil back and open to reload most of the fumes are gone.

kopyo-21 wrote:No one know it all so don't judge "meaningless" carelessly about other comments, especially when you have not really understood all what they say.

If you know about the differences beetwen the gas-operated guns (like 2A42) and recoil-operated guns (like 2A72), you will know what does the recoil 60KN of 2A72 mean.

The guns use long-recoil to operate so it neede enough the recoil force to operate the gun's mechanism instead of eliminating it. That is the reason why we have never ever seen it with muzzle brake like 2A42 and other guns for reducing the recoil force. When firing, most of 60KN recoil force will be used to power the gun system so the recoil left on the turret is very small (6KN as my source mentioned)

In the opposite way, the 2A42 guns mainly use the gas from the cartridge power burning to operate the gun system.Its recoil (without muzzle brake - I think) according to Tula's is 50-60KN. While my source mention 30-40KN (with muzzle brake that reportely help to reduce 1/3 recoil force). When firing, most of recoil force (30-40KN) will stress on the turret finally.

You don't have any sources for any of this. You are putting your own assumptions between what the manufacturer says and what a random website says and making your own conclusions. Your source also says that the 2A72 has a muzzle brake and the website has not been updated in 4 years. Most of the links are dead. Don't you think that it's far more likely that they simply made a typo and forgot to put a 0 in front of the 6?

Recoil force isn't there to "power the gun system". The gun system produces the recoil force. It is the energy from the 30mm cartridge that powers the recoil system of both guns, and the amount of energy is the same for both. Following your logic, the recoil force of both guns should be 60 kN, but it is not. That's because 60 kN is not referring to the energy of the cartridge, or the recoil force of the barrel at the moment it begins to roll back. It's the peak recoil force measured at the cannon mount. If a 30x165mm cartridge was put in a fixed barrel with no muzzle brake and a fixed breech, the recoil force (derivative of the change in momentum of the 0.4 kg bullet as it accelerates from 0 m/s to 960 m/s + the thrust from the propellant gasses at the muzzle) would be much higher than 60 kN. You can calculate this yourself: 0.4 kg projectile goes from 0 m/s to 960 m/s in a 2.416 meter barrel. Acceleration is 190,728 m/s^2. Recoil force is therefore 0.4 kg x 190728 m/s^2, so 76,291 N, or 76.3 kN. I did this on a sheet of paper, so it's probably not that accurate, but the margin of error is not enough to turn 76.3 into a 60. Of course, I don't know the velocity of the escaping propellant gasses, so I have no idea how much recoil force is produced from that, but you get the idea.

By your logic, 60kN of force is produced at the moment that the shell leaves the barrel of a 2A72, and the recoil force gets used up by the long stroke recoil action until only 6 kN is left. However, Tulamash says that the recoil force for the 2A38M is 62 kN and the recoil force for GSh-6-30K is 70 kN (even though MV of 30mm shell is less: 890 m/s vs 960 m/s). This is only possible if the recoil force is 76.3 kN, more or less, and not if the recoil force is 60 kN or less. Where does the extra ~2 kN and ~10 kN come from? The guns cannot have more energy than the cartridges can provide, even if you ignore the recoil force from the escaping gasses, so either you misunderstood the concept of recoil forces and you are ignoring what the manufacturer of the 2A72 + the technical manual says, or a 0.4 kg projectile with an MV of 890 m/s can produce 10 kN more recoil force than a 0.4 kg projectile with an MV of 960 m/s. Which do you think makes more sense? Which do you think follows the laws of physics?

Now that that's out of the way, the more important question comes up: Why would the BMPT - a heavy vehicle that should have maximum anti-infantry firepower and does not need to be concerned with poisonous fumes - need to have its dual 2A42s replaced with 2A72s that fire slower and may have worse accuracy? What requirements is this suggestion based on?

kopyo-21 wrote:
Besides bigger and much stronger recoil, one more thing important about 2A42. Its design make it be installed a little bit off right of turret axis. When firing at fast mode and long busts, it makes the turret spin and of cource, firing inaccuracy.

This does not matter for a BMPT because both 2A42 cannons are installed symmetrically in the center of the turret.

kopyo-21 wrote:
"The autocannon is mounted slightly off to the right, which causes an unfortunate tendency for the turret to be pushed slightly to the right when the autocannon fires bursts in the high rate of fire mode. The effect is totally negligible if the gunner uses short bursts, so this idiosyncrasy shouldn't pose any harmful effects to accuracy when accuracy matters most. However, the huge recoil forces generated even when firing in the low rate of fire is a tremendous burden on the horizontal stabilizer if the turret were spinning while firing. "

and

"This problem possibly led to the use of the  low-recoil 2A72 cannon in the BPPU-1 turret for the BTR-80A and the BTR-82A instead of the 2A42."

https://thesovietarmourblog.blogspot.com/2016/05/bmp-2.html?m=1
https://thesovietarmourblog.blogspot.com/2015/10/?m=1

You gave two links to my blog, but only quoted from one. If you looked in the first link, you can see that the entries for the 2A42 are different. That is because the traffic to the BMP-2 article is higher, so I prioritized updating it over the BMD-2 article. The BMD-2 article is currently outdated and needs renovation, and it's pretty obvious from the second quote:

"This problem possibly led to the use of the  low-recoil 2A72 cannon in the BPPU-1 turret for the BTR-80A and the BTR-82A instead of the 2A42."

There is no such thing as a BPPU-1 turret, and the BTR-80A and BTR-82A use different turrets. However, I can't be bothered to change that right now.

We are talking about either 2A42 or 2A72 on BTR-82A and Tigr, not only about BMPT-72.

There are two ways to talk about the gun recoil. One is the recoil that gun produces when firing. The other is the recoil that push on the gun mounting when firing. Therefore we see the differences between Tula's and Weaponsystem's data about the recoil of 2A42 and 2A72 gun.

It is very simple and no need to talk too long & too much. The 2A42 and the 2A72 fire the same rounds, have the same barrel length and barrel design so basically, according to Newton, they will produce the same recoil force. According to Tula's data, the 2A42 guns produce less recoil than 2A72. This simply because of muzzle brake on 2A42 but 2A72.

However, the differences of gun operations (gas vs recoil) make the final recoil on the turret differently. In the gas-operated guns, the most of produced recoil pushes on the gun mounting. In the long recoil operated guns, the most of produced recoil is spent to power the gun mechanism so just small left pushes on the gun mounting. That is reason to explain why they can put the 2A72 gun on the roof-top of Tigr-M.

"the long and relatively soft recoil push puts less strain on the mechanism and makes accuracy easier to achieve while avoiding the need for the whole gun to recoil in its mounting"

https://www.bevfitchett.us/heavy-machine-guns/longrecoil-mechanisms.html

kopyo-21 wrote:We are talking about either 2A42 or 2A72 on BTR-82A and Tigr, not only about BMPT-72.

There are two ways to talk about the gun recoil. One is the recoil that gun produces when firing. The other is the recoil that push on the gun mounting when firing. Therefore we see the differences between Tula's and Weaponsystem's data about the recoil of 2A42 and 2A72 gun.

It is very simple and no need to talk too long & too much. The 2A42 and the 2A72 fire the same rounds, have the same barrel length and barrel design so basically, according to Newton, they will produce the same recoil force. According to Tula's data, the 2A42 guns produce less recoil than 2A72. This simply because of muzzle brake on 2A42 but 2A72.

Ah, so the 40-50 kN recoil force of the 2A42 is less than the 60 kN of the 2A72 because of the muzzle brake. So please explain why Tulamash states that the 2A38M has more recoil force than both the 2A42 and 2A72. Only the 2A42 has a muzzle brake, so they should all have 60 kN of recoil force. They don't. How does this fit into your narrative?

At the end of the day, yes, it is very simple indeed. You have given 0 sources, despite touting "facts and figures". When I found your source for you, claimed that they represented the recoil force on the turret, when in fact it never said anything except "Recoil". What you are doing here is projecting your own assumptions into numbers given by a defunct website that has known errors in the same page as your facts, and then you build your entire narrative on those assumptions. Instead of simply accepting the facts shown to you, you created your own.

kopyo-21 wrote:However, the differences of gun operations (gas vs recoil) make the final recoil on the turret differently. In the gas-operated guns, the most of produced recoil pushes on the gun mounting. In the long recoil operated guns, the most of produced recoil is spent to power the gun mechanism so just small left pushes on the gun mounting. That is reason to explain why they can put the 2A72 gun on the roof-top of Tigr-M.

Ever heard of the Cockerill CPWS-25? It's an unmanned turret with a M242 25mm chain gun that has been mounted to the Panhard Crab (which is much smaller than a Tigr-m) and the Sherpa Scout, which is in the same class as the Tigr-M. Recoil force of the M242 is 40 kN, same as the 2A42. What is your explanation for that? As I said, it's more nuanced than you think.

kopyo-21 wrote:
"the long and relatively soft recoil push puts less strain on the mechanism and makes accuracy easier to achieve while avoiding the need for the whole gun to recoil in its mounting"

https://www.bevfitchett.us/heavy-machine-guns/longrecoil-mechanisms.html

The only long recoil 30mm cannon that is accurate despite working on a long recoil principle is the RARDEN, and that's because it fires at a glacial rate compared to a chain gun or a gas-operated gun of the same caliber.

Very easy and simple to answer your question about the recoil produced by 2A38 gun. The first, It has no muzzle brakes on their barrels. The second, its fire barrel by barrel one barrel is off-right and the other is off-left of gun's axis that make higher recoild on gun's axis. The same reason with the 6-barrel guns, the barrels are not on the gun's axis.

According to the chief designer of the SKBM Alexander Blagonravov, it is true that the 2A72 is small less accuracy than the 2A42. However they completely solve that by attaching its support sleeve to the 2A70 barrel. There is another case. Even though without barrel's support sleeve and basing on the wheeled plaform, they manage the stabilize system to make the 2A72 gun on BTR-82A have the same firing accuracy level as the 2A42 gun on tracked BMP-2. It means if they use the barrel's sleeve support, the accuracy may excess the BMP-2's level.

Back to your comments, you still avoid admitting the true things:

1. There are two recoil parameters. One is what guns produce when firing. Other is what guns finally push on the gun mountings when firing. What the weaponsystem.net mentioned is the latter, final recoil on the gun mounting.

2. Up to gun designs, the ratios between two recoil parametters mentioned above are different.

3. The long recoil operated guns push much less stress (recoil) on the gun mounting than the gas-operated guns do.

4. Give clear technical reasons why many ligh vehicles, both in Russia and Ukraine use "inferior" 2A72 instead "superior" 2A242 instead talking about political reasons you hear rumor from somewhere.


2A42 gun:
- Recoil: 30-40KN
- Fires powerful ammunition
- High rate of fire
- Good range
- Heavy recoil
- Complex design
- Due to its significant recoil the strength requirements on the mount are high
http://weaponsystems.net/weaponsystem/II04%20-%202A42.html
2a72 gun:
- Recoil: 6KN
- Fires powerful ammunition
- Limited weight
- Low recoil impulse
- Reduced accuracy due to recoilling barrel
http://weaponsystems.net/weaponsystem/II04%20-%202A72.html

and your owned coppy in your blog: "low-recoil 2A72"
https://thesovietarmourblog.blogspot.com/2015/10/?m=1

If you want to know how other light vehicles in West can install the strong recoil guns, write producers an e-mail instead asking me.

Hi Garry,

I forgot that they can mix HEI and API in the same belt to feed the guns like A-10's gun. However, like the A-10 gun, in some cases, rounds spent wastefully.

One example of what I mentioned previoudly. HEI will be very evey effective to attake troops in behigh the walls while API just make a hole and far less effective. Another example, the max effective rang of API fire by 2A42 and 2A72 is 2,000m while HEI's is 4,000m. This means that when they attake targets outside of 2,000m by mixed HEI and API rounds, all of API rounds are harmless and wastefull.

kopyo-21 wrote:Very easy and simple to answer your question about the recoil produced by 2A38 gun. The first, It has no muzzle brakes on their barrels. The second, its fire barrel by barrel one barrel is off-right and the other is off-left of gun's axis that make higher recoild on gun's axis. The same reason with the 6-barrel guns, the barrels are not on the gun's axis.

1. No muzzle brake should mean that it would have the same 60 kN as 2A72.
   
   
2. An increase in the recoil force can be expected if the mounting axis vertical and between the two barrels, because the moment is higher, but this is not the case on the 2A38 because the mount is not "off-right" or "off-left". The gun mount is horizontal, and both barrel are inline in the horizontal plane. That is why the gun can be elevated up and down on the Tunguska and Pantsir, so no, your "easy" explanation doesn't make sense at all. Plus, you forget that the barrel is not on the axis of the 2A42 either. As you said earlier, the gun is mounted slightly off to the side.
   

kopyo-21 wrote:According to the chief designer of the SKBM Alexander Blagonravov, it is true that the 2A72 is small less accuracy than the 2A42. However they completely solve that by attaching its support sleeve to the 2A70 barrel. There is another case. Even though without barrel's support sleeve and basing on the wheeled plaform, they manage the stabilize system to make the 2A72 gun on BTR-82A have the same firing accuracy level as the 2A42 gun on tracked BMP-2. It means if they use the barrel's sleeve support, the accuracy may excess the BMP-2's level.

I have no doubt that a 2A72 with a rigid support sleeve or frame can be as accurate as a 2A42, but if they can manage to stabilize the 2A72 on the BTR-82A to have the same firing accuracy level as the 2A42 without needing a support, then they can improve the stabilizer for the 2A42 so that it is more accurate than a 2A72. Unfortunately, they can't, because that is asinine, and the manufacturer knows it:



The 2A42 is highly inaccurate when firing at the high rate of fire mode, but it is sufficiently accurate when firing in the low rate of fire mode. The 2A72 may make sense for light vehicles in this case because it cannot fire in the high rate of fire mode and it is lighter and simpler, but it does not make any sense at all for a BMPT. Why did they install 2x 2A42 in the first place? When you are firing at the high rate of fire mode, you basically don't have to care about accuracy any more. If you want accuracy, fire in single shot or in short bursts from the slow rate of fire mode. BTW, no, you can't get 1,100 RPM from 2x 2A72. That is a limitation of its long recoil operating system, not its ammunition feed. The gun basically fires at its highest possible rate. The reason why it is limited at 330 RPM is because the barrel takes a long time to recoil backwards by 330-335mm and then forward again time the gun cycles, and then it has to wait for the bolt to come forward and chamber a fresh round. When the bolt locks in place, the gun fires.

kopyo-21 wrote:
Back to your comments, you still avoid admitting the true things:

1. There are two recoil parameters. One is what guns produce when firing. Other is what guns finally push on the gun mountings when firing. What the weaponsystem.net mentioned is the latter, final recoil on the gun mounting.

2. Up to gun designs, the ratios between two recoil parametters mentioned above are different.

3. The long recoil operated guns push much less stress (recoil) on the gun mounting than the gas-operated guns do.

4. Give clear technical reasons why many ligh vehicles, both in Russia and Ukraine use "inferior" 2A72 instead "superior" 2A242 instead talking about political reasons you hear rumor from somewhere.

1. This is completely true, and it's not hard to calculate that the recoil when firing is 76.3 kN. That's the recoil force from a 0.4 kg projectile travelling at an MV of 960 m/s out of a 2.416 meter barrel, not counting the contribution by propellant gasses. It is much more than 60 kN. What you are doing is arbitrarily attaching special meanings to what a random website says.
   
   
2. This is obvious. What is not obvious is why you are telling Tulamash what they mean with their numbers.
   
   
3. I thought so too, but the numbers do not agree with this.
   
   
4. Write producers an e-mail instead asking me.
   

kopyo-21 wrote:
2A42 gun:
- Recoil: 30-40KN
- Fires powerful ammunition
- High rate of fire
- Good range
- Heavy recoil
- Complex design
- Due to its significant recoil the strength requirements on the mount are high
http://weaponsystems.net/weaponsystem/II04%20-%202A42.html
2a72 gun:
- Recoil: 6KN
- Fires powerful ammunition
- Limited weight
- Low recoil impulse
- Reduced accuracy due to recoilling barrel
http://weaponsystems.net/weaponsystem/II04%20-%202A72.html

Also from the same page:
2a72 gun:
- Muzzle brake

And there are many other errors on other pages from that website, which has not been updated in 4 years. I can completely understand it if you quoted the "6 kN" recoil force from an article written by a Russian industry expert or from a book, but a random website that has no reference list, no bibliography, no sources? Even Wikipedia has links to the sources. This has nothing.

How often will it be used to engage targets at 4km range?

More importantly most heavy calibre machine guns fire different types of rounds including standard ball and HE and armour piercing and tracer... if only every second round is effective then it is no big deal... Armour piercing rounds can help smash down walls and damage their structure by punching right through them... once the walls are partially down HE rounds are vastly more effective inside a building than splattering on the wall outside the building.

In terms of light vehicles... whether they are armoured or not, then a mix of HE and AP rounds will do rather more extensive damage than HE or AP alone.

If they are ballistically matched then the API rounds will still hit targets at 4km... they just will be travelling so slow that they wont penetrate very much armour.

In other words they stop being AP but would remain Incendiary rounds... and I personally would not want to get hit by a 30mm API round even at 4km range... it would likely still smash up the engine of a normal vehicle...

The first thing: 2A38 is double barrel gun and fire barrel by barrel. Obviously the two barrel's axis are located either on the left or on the right of gun's axis. This makes the recoil stronger on the gun's axis. The same situation may happen with the 6-barrel 30mm guns like gsh-630, etc.

The second thing: The 2A42 barrel's axis is the gun's axis. The problem on BMP-2 is they installed the gun's axis off right of the turret's axis. This may be caused by gun's design and the way they feed from the side instead of from above like on Mi-28N.

You can refuse believing on the information from my sources but you can not deny that:

1. The long-recoil operated gun like 2A72 push far less recoil on the gun mounting than gas-operated gun like 2A42.

2. The 2A72 guns is the first choice of ligh combat vehicles that are newly produced not only in Russia but also in Ukraine. The producers are not stupid (as you may think) to choose the gun that is not only "higher-recoil" (as you is trying to prove) while lower-ROF and not lighter (if adding the barrel's sleeve support) than 2A42.

3. About your owned coppy "low-recoil 2A72 gun" in your blog what you is trying to ignore or refuse now, this make me understand that you have collected information from many sources to write you blog so forgotten what you copied & past on your blog before.

4. I have the points to suggest replacing 2A42 guns by 2A72 guns on new version of BMPT . The first, 2A72 is far less recoil so will make the turret less vibration, especiall when the guns are highly mounted (like on BMPT-72) and the accuracy will be improved finally. The second, if they can improve the feeding limitation (on BMPT-72) to feed both type of rounds on each gun, they can select what round they want and fire it simultaneously at maximum 1,100 rpm, enough to deal with all of its assigned targets. The third, it is cheaper to acquire and maintain the 2A72 guns.

Your problem is you think you know all but in reality you totally don't.

So is the recoil force stated by Tulamash the recoil force of the gun, or the recoil force on the turret? As you said before, the recoil force of a gun like the 2A42 is 40-50 kN, but it gets reduced by the muzzle brake to 30-40 kN and the turret receives 30-40 kN. If that is the case, then the given recoil force from the website must be pure recoil of the gun without considering any other factors, like the nonsense that you spouted. How does your logic fit that in? You can't, because it's nonsensical and self-contradictory.

I will believe in your sources when you show them.

As for the accuracy (and lethality) of 2A72, this graph may be interesting. It is aimed at showing the advantages of the BMP-1M with the "Kliver" turret compared to a regular BMP-1. Graph taken from БМП-1 (1964-2000) by Sergey Malyshev.



Graph on the right shows the probability of destroying a generic APC with 16 rounds from the 30mm AP (autocannon) when firing from a stationary position and when firing on the move compared to 2 shots from the 73mm cannon of the BMP-1. The distance where the probability of destroying an APC with 16 rounds from a 2A72 cannon is practically guaranteed (0.8) is 1.2 km when firing from a stationary position and 1.0 km when firing on the move. Probability of destroying an APC with the "Grom" 73mm cannon with 2 shots at 500 meters is 0.8, which is why 500 m is considered the effective range of the cannon. This might be useful to someone.

The first thing: 2A38 is double barrel gun and fire barrel by barrel. Obviously the two barrel's axis are located either on the left or on the right of gun's axis. This makes the recoil stronger on the gun's axis. The same situation may happen with the 6-barrel 30mm guns like gsh-630, etc.

Recoil is important if it is too much for the platform it is mounted on... for a vehicle however most IFVs these days are well over 15 tons so 5 tons of recoil is not that big a deal.

the twin barrel 30mm 2A38M is more accurate than their 6 barrel gatlings... fixed barrels are more accurate than revolving ones, though revolving barrels can often have each barrel offset to improve or reduce accuracy.

A shotgun is effective because it delivers lots of projectiles around the point of aim so if the target changes path after the shot is fired and before the rounds impact there is still a good chance of hits against small fast targets.

1. The long-recoil operated gun like 2A72 push far less recoil on the gun mounting than gas-operated gun like 2A42.

Guns with similar barrel lengths firing the same ammo have the same recoil energy.

A gas operated rifle has less felt recoil than a bolt action, and a long recoil operated SMG has even less felt recoil because the energy is spread over a longer period.

All of this is important if you are shoulder firing the weapon but a fixed mount in a recoil absorbing system and it really does not matter at all.

4. I have the points to suggest replacing 2A42 guns by 2A72 guns on new version of BMPT . The first, 2A72 is far less recoil so will make the turret less vibration, especiall when the guns are highly mounted (like on BMPT-72) and the accuracy will be improved finally. The second, if they can improve the feeding limitation (on BMPT-72) to feed both type of rounds on each gun, they can select what round they want and fire it simultaneously at maximum 1,100 rpm, enough to deal with all of its assigned targets. The third, it is cheaper to acquire and maintain the 2A72 guns.

The choice of the 2A42 was always a compromise... it is heavy but reliable, but most importantly it is cheap.

Considering the vehicle it is actually replacing in the ground troop fire support role is actually the Tunguska and Shilka I personally think replacing the two single barrel guns with a single 2A38M cannon makes rather more sense with a rate of fire reduction and burst limiter... I mean if they are firing from fixed belts anyway why not use a weapon with a higher rate of fire in short bursts... it would also be more effective against aircraft.

I rather suspect in the near future the combination of high velocity 57mm guns and 57mm grenade launchers will largely replace the 30mm weapons and 40mm grenade launchers will totally replace the 30mm weapons in service now.

I agree that they can install 2A42 gun on light combat wheeled vehicles like BTR-82A, BTR-4, Uran-9, Tiger-M but with the trade-off of firing accuracy. If they want to keep the firing accuracy while on stop and while on move, they will have to reinforce gun mounting, turret, hull and chassis. In that case, the vehicles will gain a significant weight that may ask for changing engine, reducing the protection level, etc.

less felt recoil because the energy is spread over a longer period.

Just a minor correction, Garry. Technically speaking, felt recoil is not really about energy as in kinetic energy, but about momentum. That's why recoil force is expressed as a force, in Newtons, and not as energy, in Joules.

Also, about the "HE vs wall" thing, here's what 30x165mm HE shells do to walls, at least when they are fired out of a Finnish BMP-2, according to an ex-BMP-2 mechanic posting on Reddit.

Well since it's my first ever post on reddit I have no clue about what I'm doing here, but this is an album I compiled from my time in the Finnish Defence Forces as a BMP-2 mechanic. Actually seeing what these suckers really did to targets gave me a new respect for the 30mm cannon the BMP-2 has.

Here's his imgur album: https://imgur.com/a/zBP1K

10cm concrete brick wall reinforced with rebar:



The back of the wall:

Comparison with the gun 2A42:

The 2A72 cannon, due to the use of a moving barrel scheme, transfers to the roof of the armored object a more extended recoil momentum, which means it has a smaller maximum recoil force, which makes it possible to use it on light armored vehicles such as BTR-82, Tigr- M with armored corps made of sheets with a thickness of only about 7mm, on which the use of armament more powerful than the 14.5 mm KPVT machine gun before the appearance of the 30mm gun 2A72 was previously impossible. This puts them on the same level as the heavier and more expensive infantry combat vehicles and significantly increases the combat value of light armored vehicles in defense (when the battle is fought from the trench and the level of armor protection of the combat vehicle is not so important).

The fee for using the scheme with a movable barrel was a somewhat worse accuracy and a much smaller rate of fire - only 300-330 rds / min, which makes it impossible to effectively use this gun for air targets.

http://www.history147.ru/2017/09/blog-post.html?m=1

Just looked back the Newton formula to calculate how much force needed to stop the barrel of 2A72 gun when it moves back after firing.

V= m*v/M = 0.4*960/36 = 10.677 m/s

Notes:
V: the moving back velocity of gun's barrel.
v: the projectile's muzzle velocity (960 m/s)
M: the weigh of gun's barrel that moves back when firing (36 kg)
m: the weight of projectile (0.4 kg).
(Assumption that friction is 0 and just only the gun's barrel moves back after firing. In reality, the barrel when moving back will push some parts to power the gun's mechanism)

The force needed to stop the gun's barrel moving back:

F = 1/2*M*V*V/S = 1/2*36*10.67*10.67/0.33 = 6,210 N = 6.2 KN

Notes:
F: Force need to stop the gun's barrel.
V: the move back velocity of gun's barrel (10.67 m/s)
M: the weigh of gun's barrel that moves back when firing (36 kg).
S: The move back distance of the gun's barrel (0.33 m).

This calculation has proved the recoil 6 KN of 2A72 gun that mentioned by Weaponsystem.net is accuracy.

Using the same formula to calculate the force needed to stop the 2A42 gun's barrel moving back:
- The barrel's weight is 38.5 kg.
- The distance moving back is 30 to 35 mm.
The result (without muzzle brake factor) is around 62 KN.

I personally think the AGS 57 is a waste of time and they should just use the Vasilek instead

The Vasilek has powerful HE rounds that could demolish most structures in a few hits and it also has the ability to be used is indirect fire when needed.

It can also fire AP rounds and HEAT rounds could be made for it to give it effectiveness against enemy IFVs.

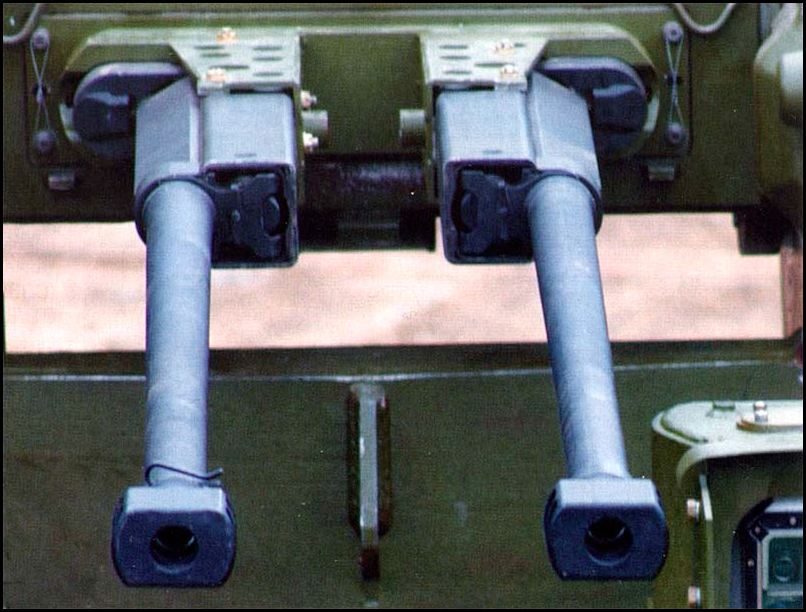
kopyo-21 wrote:Just looked back the Newton formula to calculate how much force needed to stop the barrel of 2A72 gun when it moves back after firing.

Firstly, your calculations contradict what you said earlier. Earlier, you said that 60 kN is the recoil force of the gun. 60 kN gets reduced to 6 kN by the long recoil action of the 2A72, 60 kN gets reduced to 30-40 kN by the muzzle brake of the 2A42, and 60 kN gets increased to 62 kN by the off-axis barrels in the 2A38. Why is there no 60 kN anywhere in your calculations? In your first line of calculation that the recoil force is already more than 60 kN.


V= m*v/M = 0.4*960/36 = 10.677 m/s

a=v^2/2m
a=190,728 m/s^2

Where:
v: the projectile's muzzle velocity (960 m/s)
m: the weight of projectile (0.4 kg).

F=ma

F=0.4*190,728 m/s^2 = 76.3 kN

Secondly, your math is technically correct in the simplest of terms, but you ignored too many factors for your calculations to be comparable to the real thing. The first clue is that 60 kN is nowhere present in your calculations, neither at the beginning of the shot or when the recoiling parts are stopped. The Russian MoD and Tulamash are not spreading fake news in their technical documents and websites.

1. The reality is that the barrel is stopped at a distance of 330-335mm, but it moves back under the small resistance of the weak return spring of the bolt for a distance of 270mm, and is stopped at 60-65mm by the strong shock absorber spring wrapped around the base of the barrel. The majority of the load is on the shock absorber, and it is the shock absorber spring that pushes the barrel back into battery, not the return spring which is just there to return the bolt back into battery. This is clearly mentioned in the technical description of the 2A72.
   
   
2. Furthermore, you failed to account for the thrust from the propellant gasses that contribute a huge amount to the recoil force, which cannot be calculated by the momentum of the bullet/shell alone. The propellant charge in a 30x165mm cartridge weighs 0.12 kg. The exact peak velocity of those escaping gasses at the muzzle depends on the case volume, barrel length, pressure at the muzzle, density of the propellant, atmospheric pressure, and so on, but generally speaking, the velocity is 1.5 times that of the bullet or cannon shell according to the Textbook of Small Arms. In that case, the velocity is 1920 m/s. The gasses don't all come out at this velocity due to the pressure curve, so it's not possible to accurately calculate the recoil force from the momentum of the gasses as easily as bullets and cannon shells, but it is very high indeed. If you get momentum from the mass of the propellant (0.12 kg) and its peak velocity (1920 m/s), you will find that it is 2/3 the amount of the shell (230 kg.m/s vs 384 kg.m/s), but in real life it's a bit lower because of the pressure curve mentioned earlier.
   

Consider these two factors in your calculations and your answer will definitely be much closer to 60 kN than 6 kN. Of course, there are more variables and nuances in the design of the cannon, so don't expect to get a very accurate answer.

Your calculations for the 2A42 are invalid because the momentum doesn't all go into the recoiling barrel. You forgot that there is a bolt assembly with a recoil spring that works using the energy of the propellant gasses (calculate with pressure in this case, not momentum), and the recoil force from the bolt decelerating inside the cannon receiver was ignored. It may not be obvious, but the combination of recoil reduction techniques used for the 2A42 are much more effective than you think. It combines a recoiling barrel with a large muzzle brake plus a reciprocating action, which is used on some large caliber sniper rifles because of its excellent recoil reduction characteristics. See the Barrett M82 as an example. It's got a large double-baffle muzzle brake (reduces felt recoil by 70% according to Sniper: A History of the US Marksman) like the 2A42, a barrel that recoils back (by 1 inch) with each shot like the 2A42 and a reciprocating bolt that further softens the felt recoil like the 2A42.



If you believe that the recoil force of the 2A42, 2A72 and 2A38 are 40-50 kN, 60 kN and 62 kN respectively and the recoil force of 30x165mm from a static barrel is much higher than 60 kN, then it all makes sense. For example, the recoil force from a McMillan TAC-50 with no muzzle brake is 33 kN, and that's a .50 caliber round. That's slightly more than half of the 60 kN for a 30mm round that you claimed, and 62 kN without muzzle brake as you calculated just now. Also, the recoil force from the M242 25mm cannon is 40 kN. So a 25mm cannon has only 20% more recoil force than a .50 caliber rifle and a 30mm cannon has only 2x as much recoil force? That's absurd, but that's exactly what it means if we follow your logic.

Consider this. These numbers show the felt recoil from these guns:
2A42: 40-50 kN
2A72: 60 kN
2A38: 62 kN
M242: 40 kN
TAC-50: 2.3 kN

Calculated recoil force from bullet alone fired from fixed barrel, no muzzle brake:
12.7x99mm (from 0.686 m barrel): 19.8 kN

33/19.8=1.67
So the recoil force from the propellant in a TAC-50 increases the recoil force by 1.67 times compared to just the recoil force from the bullet only. Apply the same multiplier to the 30 and 25mm rounds and you get 127 kN and 89.5 kN. Not the most scientific way to do things, but we lack the information to be more precise.

So the recoil of a .50 caliber round is 33 kN, and the recoil of a 30mm round is around 120 kN. The felt recoil of the TAC-50 is only 2.3 kN despite a .50 caliber round having 33 kN of recoil force is due to a huge and quite advanced quadruple-baffle muzzle brake combined with a special hydraulic recoil dampener, and the felt recoil of the 30mm round is reduced from around 120 kN to between 40-62 kN depending on the gun that fires it. This makes a lot more sense than what you are proposing, not to mention that it corresponds to real facts from other examples.

PS: Why does the 2A42 have almost as little recoil as an M242 even though both have muzzle brakes? Because the barrel of the 2A42 recoils by 30-35mm and there is a bolt assembly with a recoil spring inside the cannon, whereas the M242 has a fixed barrel and no reciprocating parts since it's an electrically powered chain gun. All it has is a muzzle brake. More recoil, but more accurate. This is contrary to what you said about "low recoil force = higher accuracy" because there are much more important factors to accuracy than recoil force.

Why does the 2A38 have only a little more recoil than a long recoil cannon like the 2A72? 2A38 is a two barrel gun that works on the Gast principle, meaning that the recoil from one barrel operates the action of the other barrel. In this case, the gasses from the first barrel pushes first bolt assembly backwards and compresses the recoil spring, and the first bolt assembly pushes the second bolt assembly forward using a rack and pinion system. This means that the recoil from one shot is being absorbed by two large recoil springs: one is being compressed, and one is being extended. Also, the mass of the two bolts go in opposite directions, so a part of the recoil forces are cancelled out (like in the Russian counter-recoil rifles). This adequately explains why the recoil force is halved compared to the calculated 127 kN.

Hopefully that explains everything for you.